Trong mặt phẳng $Oxy$, cho các vectơ $\overrightarrow{a}=(0;1)$, $\overrightarrow{b}=(-1;2)$, $\overrightarrow{c}=(-3;-2)$. Tọa độ của vectơ $\overrightarrow{u}=3\overrightarrow{a}+2\overrightarrow{b}-4\overrightarrow{c}$ là
| $(10;-15)$ | |
| $(15;10)$ | |
| $(10;15)$ | |
| $(-10;15)$ |
Trong mặt phẳng $Oxy$, cho hai vectơ $\overrightarrow{a}=(2;-4)$, $\overrightarrow{b}=(-5;3)$. Tìm tọa độ của vectơ $\overrightarrow{x}=2\overrightarrow{a}-\overrightarrow{b}$.
| $\overrightarrow{x}=(7;-7)$ | |
| $\overrightarrow{x}=(9;5)$ | |
| $\overrightarrow{x}=(9;-11)$ | |
| $\overrightarrow{x}=(-1;5)$ |
Trong mặt phẳng $Oxy$, cho $\overrightarrow{a}=(3;-4)$, $\overrightarrow{b}=(-1;2)$. Tọa độ của $\overrightarrow{a}+\overrightarrow{b}$ là
| $(-4;6)$ | |
| $(2;-2)$ | |
| $(4;-6)$ | |
| $(-3;-8)$ |
Trong mặt phẳng $Oxy$, cho hai vectơ $\overrightarrow{a}=\left(3;-4\right)$ và $\overrightarrow{b}=\left(1;-2\right)$. Tìm tọa độ của vectơ $\overrightarrow{a}+\overrightarrow{b}$.
| $\left(2;-2\right)$ | |
| $\left(4;-6\right)$ | |
| $\left(4;6\right)$ | |
| $\left(-4;6\right)$ |
Trong mặt phẳng $Oxy$, vectơ $\overrightarrow{a}=-9\overrightarrow{i}+4\overrightarrow{j}$ có tọa độ là
| $(4;-9)$ | |
| $\left(-9\overrightarrow{i};4\overrightarrow{j}\right)$ | |
| $(-9;4)$ | |
| $\left(-\overrightarrow{i};\overrightarrow{j}\right)$ |
Cho $\overrightarrow{a}=\left(6;5\right)$, $\overrightarrow{b}=\left(3;-2\right)$. Tìm tọa độ $\overrightarrow{c}$ sao cho $2\overrightarrow{a}+3\overrightarrow{c}=\overrightarrow{b}$.
| $\overrightarrow{c}=\left(-3;-4\right)$ | |
| $\overrightarrow{c}=\left(3;-4\right)$ | |
| $\overrightarrow{c}=\left(-2;-3\right)$ | |
| $\overrightarrow{c}=\left(-3;-2\right)$ |
Trong mặt phẳng $Oxy$, cho $\overrightarrow{u}=(3;-2)$, $\overrightarrow{v}=(7;4)$. Tìm tọa độ của $\overrightarrow{x}=3\overrightarrow{u}-4\overrightarrow{v}$.
| $\overrightarrow{x}=(19;22)$ | |
| $\overrightarrow{x}=(-19;-22)$ | |
| $\overrightarrow{x}=(-19;22)$ | |
| $\overrightarrow{x}=(19;-22)$ |
Trong mặt phẳng $Oxy$, cho vectơ $\overrightarrow{u}=2\overrightarrow{j}-5\overrightarrow{i}$. Tọa độ của $\overrightarrow{u}$ là
| $\overrightarrow{u}=(-5;2)$ | |
| $\overrightarrow{u}=(2;-5)$ | |
| $\overrightarrow{u}=(5;2)$ | |
| $\overrightarrow{u}=(2;5)$ |
Trong mặt phẳng \(Oxy\), cho ba điểm \(A(1;3)\), \(B(-1;2)\) và \(C(-2;1)\). Tìm tọa độ vectơ \(\overrightarrow{AB}-\overrightarrow{AC}\).
| \(\overrightarrow{AB}-\overrightarrow{AC}=(-5;-3)\) | |
| \(\overrightarrow{AB}-\overrightarrow{AC}=(1;1)\) | |
| \(\overrightarrow{AB}-\overrightarrow{AC}=(-1;2)\) | |
| \(\overrightarrow{AB}-\overrightarrow{AC}=(-1;1)\) |
Trong mặt phẳng tọa độ \(Oxy\), cho hai vectơ \(\vec{u}=(-1;2)\) và \(\vec{v}=(3;-2)\). Tính tọa độ của vectơ \(2\vec{u}-3\vec{v}\).
| \((11;-10)\) | |
| \((9;-10)\) | |
| \((-11;-2)\) | |
| \((-11;10)\) |
Trong mặt phẳng \(Oxy\), cho hai vectơ \(\vec{a}=(2;-4)\) và \(\vec{b}=(-5;3)\). Tìm tọa độ vectơ $\vec{u}=2\vec{a}-\vec{b}$.
| \(\vec{u}=(7;-7)\) | |
| \(\vec{u}=(9;-11)\) | |
| \(\vec{u}=(9;-5)\) | |
| \(\vec{u}=(-1;5)\) |
Trong mặt phẳng \(Oxy\), cho hai vectơ \(\vec{a}=(-1;2)\) và \(\vec{b}=(5;-7)\). Tìm tọa độ vectơ $\vec{w}=\vec{a}-\vec{b}$.
| \(\vec{w}=(6;-9)\) | |
| \(\vec{w}=(4;-5)\) | |
| \(\vec{w}=(-6;9)\) | |
| \(\vec{w}=(-5;-14)\) |
Trong mặt phẳng \(Oxy\), cho hai vectơ \(\vec{a}=(3;-4)\) và \(\vec{b}=(-1;2)\). Tìm tọa độ vectơ $\vec{v}=\vec{a}+\vec{b}$.
| \(\vec{v}=(-4;6)\) | |
| \(\vec{v}=(2;-2)\) | |
| \(\vec{v}=(4;-6)\) | |
| \(\vec{v}=(-3;-8)\) |
Trong mặt phẳng $Oxy$, cho hai đường thẳng song song $d\colon2x-3y-1=0$ và $d'\colon2x-3y+5=0$. Phép tịnh tiến theo vectơ nào sau đây không thể biến $d$ thành $d'$?
| $\overrightarrow{u}=(0;2)$ | |
| $\overrightarrow{u}=(-3;0)$ | |
| $\overrightarrow{u}=(3;4)$ | |
| $\overrightarrow{u}=(-1;1)$ |
Trong mặt phẳng $Oxy$, cho đường thẳng $d\colon2x-y+1=0$. Để phép tịnh tiến theo vectơ $\overrightarrow{v}$ biến $d$ thành chính nó thì $\overrightarrow{v}$ có thể là vectơ nào sau đây?
| $\overrightarrow{v}=(2;1)$ | |
| $\overrightarrow{v}=(2;-1)$ | |
| $\overrightarrow{v}=(1;2)$ | |
| $\overrightarrow{v}=(-1;2)$ |
Trong mặt phẳng $Oxy$, cho hai đường thẳng song song $d\colon x+y+1=0$ và $d'\colon x+y-1=0$. Biết rằng phép tịnh tiến $\mathrm{T}_{\overrightarrow{v}}$ biến đường thẳng $d$ thành đường thẳng $d'$ và vectơ $\overrightarrow{v}$ cùng phương với vectơ đơn vị $\overrightarrow{i}$. Hãy tìm tọa độ vectơ $\overrightarrow{v}$.
| $\overrightarrow{v}=(2;0)$ | |
| $\overrightarrow{v}=(0;2)$ | |
| $\overrightarrow{v}=(0;-2)$ | |
| $\overrightarrow{v}=(-2;0)$ |
Cho lưới tọa độ như hình vẽ.
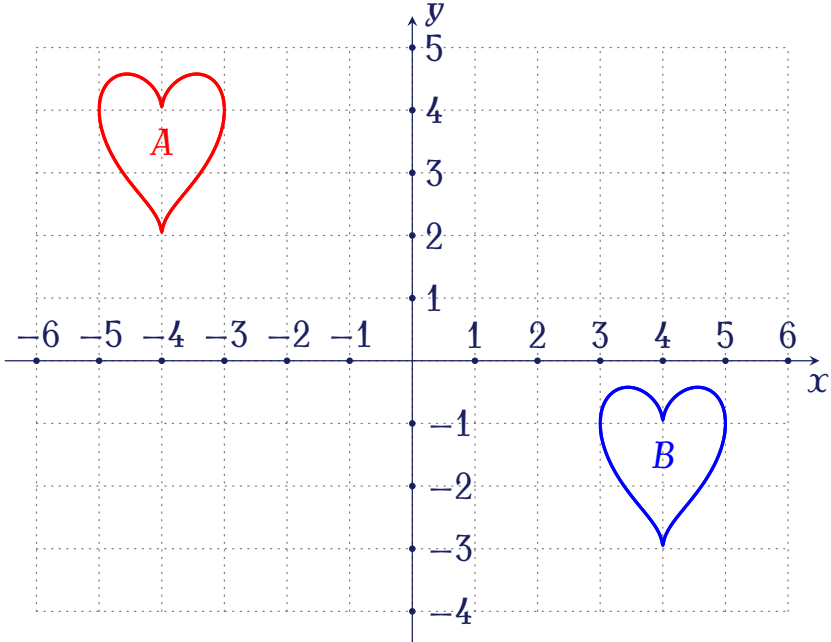
Tìm tọa độ vectơ $\overrightarrow{v}$ biết rằng phép tịnh tiến $\mathrm{T}_{\overrightarrow{v}}$ biến hình $A$ thành hình $B$.
| $\overrightarrow{v}=(8;-5)$ | |
| $\overrightarrow{v}=(-8;5)$ | |
| $\overrightarrow{v}=(8;-3)$ | |
| $\overrightarrow{v}=(8;3)$ |
Cho lưới tọa độ như hình vẽ.
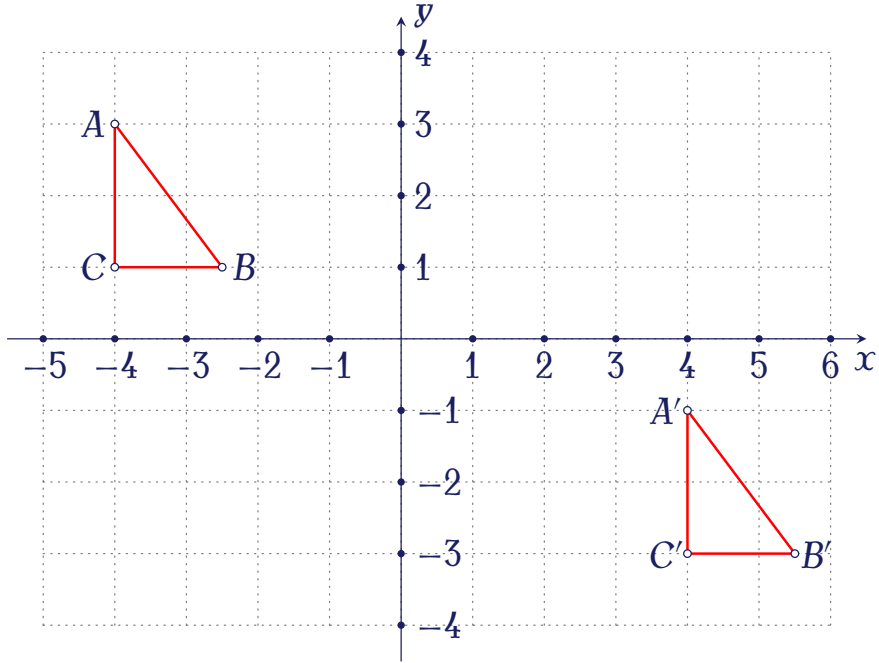
Tìm tọa độ vectơ $\overrightarrow{v}$ biết rằng phép tịnh tiến $\mathrm{T}_{\overrightarrow{v}}$ biến tam giác $ABC$ thành tam giác $A'B'C'$.
| $\overrightarrow{v}=(8;-4)$ | |
| $\overrightarrow{v}=(-8;4)$ | |
| $\overrightarrow{v}=(8;-3)$ | |
| $\overrightarrow{v}=(8;3)$ |
Biết rằng phép tịnh tiến theo vectơ $\overrightarrow{v}$ biến điểm $A(1;3)$ thành điểm $A'(1;7)$. Tìm tọa độ vectơ $\overrightarrow{v}$.
| $(0;-4)$ | |
| $(4;0)$ | |
| $(0;4)$ | |
| $(0;5)$ |
Trong mặt phẳng $Oxy$, gọi $N(2;1)$ là ảnh của điểm $M(1;-2)$ qua phép tịnh tiến $\mathrm{T}_{\overrightarrow{u}}$. Tọa độ vectơ $\overrightarrow{u}$ là
| $(1;-3)$ | |
| $(-1;3)$ | |
| $(3;-1)$ | |
| $(1;3)$ |
