Tính tích phân \(I=\displaystyle\int\limits_1^{\ln3}\dfrac{1}{e^x}\mathrm{\,d} x.\)
| \(\dfrac{1}{e-2}\) | |
| \(\dfrac{3-e}{3e}\) | |
| \(3e^{-1}\) | |
| \(e^2-2\) |
Cho hàm số bậc bốn $y=f(x)$. Biết rằng hàm số $g(x)=\ln f(x)$ có bảng biến thiên như sau:
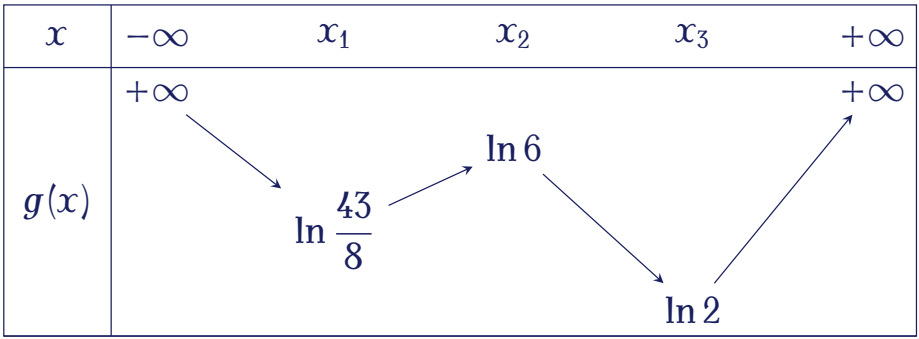
Diện tích hình phẳng giới hạn bởi các đường $y=f'(x)$ và $y=g'(x)$ thuộc khoảng nào dưới đây?
| $(5;6)$ | |
| $(4;5)$ | |
| $(2;3)$ | |
| $(3;4)$ |
Xét tích phân $I=\displaystyle\displaystyle\int\limits_1^{\rm{e}^2}\dfrac{\left(1+2\ln x\right)^2}{x}\mathrm{\,d}x$, nếu đặt $t=1+2\ln{x}$ thì $I$ bằng
| $\dfrac{1}{2}\displaystyle\displaystyle\int\limits_1^{e^2}t^2\mathrm{\,d}t$ | |
| $2\displaystyle\displaystyle\int\limits_1^5t^2\mathrm{\,d}t$ | |
| $2\displaystyle\displaystyle\int\limits_1^{e^2}t^2\mathrm{\,d}t$ | |
| $\dfrac{1}{2}\displaystyle\displaystyle\int\limits_1^5t^2\mathrm{\,d}t$ |
Tích phân $\displaystyle\displaystyle\int\limits_{0}^{10}x\mathrm{e}^{30x}\mathrm{\,d}x$ bằng
| $\dfrac{1}{900}\left(299\mathrm{e}^{300}+1\right)$ | |
| $300-900\mathrm{e}^{300}$ | |
| $-300+900\mathrm{e}^{300}$ | |
| $\dfrac{1}{900}\left(299\mathrm{e}^{300}-1\right)$ |
Xét hàm số $f(x)=\mathrm{e}^x+\displaystyle\int\limits_{0}^{1}xf(x)\mathrm{\,d}x$. Giá trị $f\left(\ln5620\right)$ bằng
| $5622$ | |
| $5620$ | |
| $5618$ | |
| $5621$ |
Tính tích phân $I=\displaystyle\displaystyle\int\limits_{0}^{1}\left(3x^2+\mathrm{e}^x+\dfrac{1}{x+1}\right)\mathrm{d}x$.
Bằng cách đổi biến số $t=1+\ln x$ thì tích phân $\displaystyle\displaystyle\int\limits_1^\mathrm{e}\dfrac{(1+\ln x)^2}{x}\mathrm{\,d}x$ trở thành
| $\displaystyle\displaystyle\int\limits_1^\mathrm{e}t^2\mathrm{\,d}t$ | |
| $\displaystyle\displaystyle\int\limits_1^2t^2\mathrm{\,d}t$ | |
| $\displaystyle\displaystyle\int\limits_1^4t^2\mathrm{\,d}t$ | |
| $\displaystyle\displaystyle\int\limits_1^2(1+t)^2\mathrm{\,d}t$ |
Cho hàm số $y=2^x$ có đồ thị là đường cong trong hình bên.
Diện tích $S$ của hình phẳng được tô đậm trong hình bằng
| $S=\displaystyle\displaystyle\int\limits_{1}^{2}2^x\mathrm{\,d}x$ | |
| $S=\displaystyle\displaystyle\int\limits_{0}^{2}2^{2x}\mathrm{\,d}x$ | |
| $S=\pi\displaystyle\displaystyle\int\limits_{0}^{2}2^x\mathrm{\,d}x$ | |
| $S=\displaystyle\displaystyle\int\limits_{0}^{2}2^x\mathrm{\,d}x$ |
Biết $\displaystyle\displaystyle\int\limits_{-1}^1\left(\dfrac{9}{x-3}-\dfrac{7}{x-2}\right)\mathrm{\,d}x=a\ln{3}-b\ln{2}$. Tính giá trị $P=a^2+b^2$.
| $P=32$ | |
| $P=130$ | |
| $P=2$ | |
| $P=16$ |
Diện tích hình phẳng giới hạn bởi đồ thị hàm số $y=\mathrm{e}^x$ và các đường thẳng $y=0$, $x=0$, $x=2$ bằng
| $\pi\displaystyle\displaystyle\int\limits_{0}^{2}\mathrm{e}^x\mathrm{\,d}x$ | |
| $\displaystyle\displaystyle\int\limits_{0}^{2}\mathrm{e}^{2x}\mathrm{\,d}x$ | |
| $\pi\displaystyle\displaystyle\int\limits_{0}^{2}\mathrm{e}^{2x}\mathrm{\,d}x$ | |
| $\displaystyle\displaystyle\int\limits_{0}^{2}\mathrm{e}^x\mathrm{\,d}x$ |
Biết $\displaystyle\displaystyle\int\limits_{0}^{2}(3x-1)\mathrm{e}^{\tfrac{x}{2}}\mathrm{\,d}x=a+b\mathrm{e}$ với $a,\,b$ là các số nguyên. Giá trị của $a+b$ bằng
| $12$ | |
| $16$ | |
| $6$ | |
| $10$ |
Cho hàm số $y=f(x)$ liên tục trên khoảng $(0;+\infty)$. Biết $f(1)=1$ và $f(x)=xf'(x)+\ln x$, $\forall x\in(0;+\infty)$. Giá trị của $f(\mathrm{e})$ bằng
| $\mathrm{e}$ | |
| $\dfrac{1}{\mathrm{e}}$ | |
| $1$ | |
| $2$ |
Biết rằng \(I=\displaystyle\int\limits_{1}^{a}\dfrac{\ln x}{x^2}\mathrm{\,d}x=\dfrac{1-\ln2}{2}\). Giá trị của \(a\) bằng
| \(2\) | |
| \(\ln2\) | |
| \(4\) | |
| \(8\) |
Tính tích phân \(I=\displaystyle\int\limits_{1}^{2}x\left(\mathrm{e}^x-\dfrac{1}{x}\right)\mathrm{\,d}x\).
| \(I=\mathrm{e}^2-1\) | |
| \(I=\mathrm{e}^2\) | |
| \(I=\mathrm{e}^2+1\) | |
| \(I=\mathrm{e}^2-2\) |
Tích phân \(I=\displaystyle\int\limits_{0}^{1}x\cdot2^x\mathrm{\,d}x\) bằng
| \(\dfrac{2\ln2-1}{\ln^22}\) | |
| \(\dfrac{2\ln2-1}{\ln2}\) | |
| \(\dfrac{2\ln2+1}{\ln^22}\) | |
| \(\dfrac{2\ln2+1}{\ln2}\) |
Tính tích phân \(I=\displaystyle\int\limits_{1}^{\mathrm{e}}\dfrac{\sqrt{2+\ln x}}{2x}\mathrm{\,d}x\).
| \(\dfrac{3\sqrt{3}+2\sqrt{2}}{3}\) | |
| \(\dfrac{\sqrt{3}+\sqrt{2}}{3}\) | |
| \(\dfrac{\sqrt{3}-\sqrt{2}}{3}\) | |
| \(\dfrac{3\sqrt{3}-2\sqrt{2}}{3}\) |
Biết \(\displaystyle\int\limits_{1}^{2}\dfrac{\mathrm{d}x}{(x+1)(2x+1)}=a\ln2+b\ln3+c\ln5\). Khi đó giá trị \(a+b+c\) bằng
| \(1\) | |
| \(0\) | |
| \(2\) | |
| \(-3\) |
Xét \(\displaystyle\int\limits_0^2x\cdot\mathrm{e}^{x^2}\mathrm{\,d}x\), nếu đặt \(u=x^2\) thì \(\displaystyle\int\limits_0^2x\cdot\mathrm{e}^{x^2}\mathrm{\,d}x\) bằng
| \(2\displaystyle\int\limits_0^2\mathrm{e}^u\mathrm{\,d}u\) | |
| \(2\displaystyle\int\limits_0^4\mathrm{e}^u\mathrm{\,d}u\) | |
| \(\dfrac{1}{2}\displaystyle\int\limits_0^2\mathrm{e}^u\mathrm{\,d}u\) | |
| \(\dfrac{1}{2}\displaystyle\int\limits_0^4\mathrm{e}^u\mathrm{\,d}u\) |
Hàm số \(y=f(x)\) liên tục trên \([1;4]\) và thỏa mãn \(f(x)=\dfrac{f\left(2\sqrt{x}-1\right)}{\sqrt{x}}+\dfrac{\ln x}{x}\). Tính tích phân \(I=\displaystyle\int\limits_{3}^{4}f(x)\mathrm{\,d}x\).
| \(I=3+2\ln^22\) | |
| \(I=\ln^2\) | |
| \(I=2\ln2\) | |
| \(I=2\ln^22\) |
Cho \(\displaystyle\int\limits_{0}^{1}\dfrac{x^2+1}{x+1}\mathrm{\,d}x=a+b\ln c\), với \(a\in\mathbb{Q}\), \(b\in\mathbb{Z}\), \(c\) là số nguyên tố. Ta có \(2a+b+c\) bằng
| \(5\) | |
| \(4\) | |
| \(3\) | |
| \(2\) |
