Ngân hàng bài tập
C
Gọi \(S\) là diện tích hình phẳng giới hạn bởi đồ thị hàm số \(y=f(x)\), trục hoành, \(x=a\), \(x=b\).
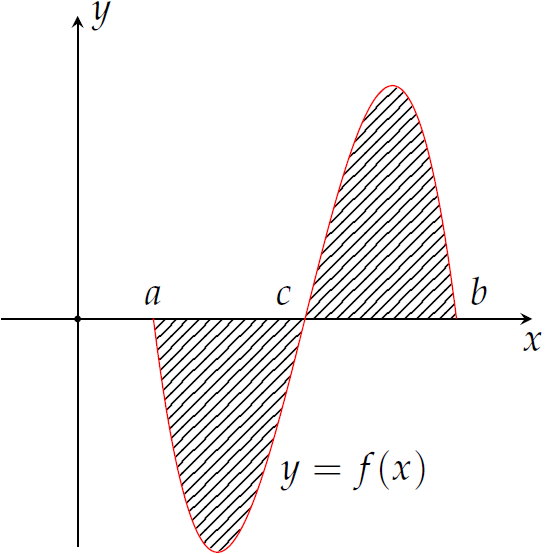
Khi đó \(S\) được tính theo công thức nào dưới đây?
| \(S=\displaystyle\int\limits_{a}^{b}f(x)\mathrm{\,d}x\) | |
| \(S=\displaystyle\int\limits_{a}^{c}f(x)\mathrm{\,d}x+\displaystyle\int\limits_{c}^{b}f(x)\mathrm{\,d}x\) | |
| \(S=-\displaystyle\int\limits_{a}^{c}f(x)\mathrm{\,d}x+\displaystyle\int\limits_{c}^{b}f(x)\mathrm{\,d}x\) | |
| \(S=\left|\displaystyle\int\limits_{a}^{c}f(x)\mathrm{\,d}x+\displaystyle\int\limits_{c}^{b}f(x)\mathrm{\,d}x\right|\) |
1 lời giải
Chọn phương án C.
\(S=\displaystyle\int\limits_{a}^{b}\left|f(x)\right|\mathrm{\,d}x=-\displaystyle\int\limits_{a}^{c}f(x)\mathrm{\,d}x+\displaystyle\int\limits_{c}^{b}f(x)\mathrm{\,d}x\).
