Ngân hàng bài tập
A
Tìm số nghiệm nguyên của bất phương trình $$2^{x+2}+8\cdot2^{-x}-33<0$$
| \(4\) | |
| \(6\) | |
| \(7\) | |
| Vô số |
1 lời giải
Chọn phương án A.
Đặt \(t=2^x\;(t>0)\), ta có $$\begin{aligned}
&\,2^{x+2}+8\cdot2^{-x}-33<0\\
\Leftrightarrow&\,4\cdot2^x+\dfrac{8}{2^x}-33<0\\
\Leftrightarrow&\,4\cdot\left(2^x\right)^2-33\cdot2^x+8<0\\
\Leftrightarrow&\,4t^2-33t+8<0.
\end{aligned}$$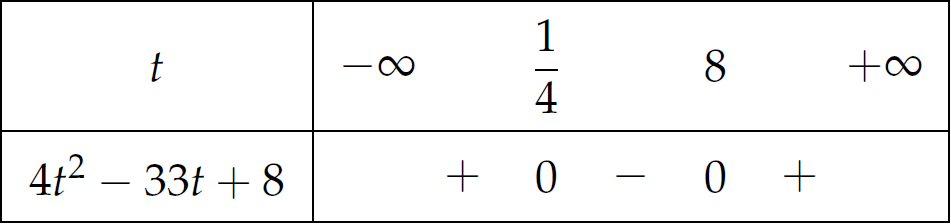
Suy ra $$\begin{aligned}
\begin{cases}
t>\dfrac{1}{4}\\ t<8
\end{cases}\Leftrightarrow&\begin{cases}
2^x>\dfrac{1}{4}\\ 2^x<8
\end{cases}\\
\Leftrightarrow&\begin{cases}
x>-2\\ x<3.
\end{cases}
\end{aligned}$$
Vậy phương trình đã cho có \(4\) nghiệm nguyên là \(-1;0;1;2\).
