Ngân hàng bài tập
B
Tìm tập nghiệm của bất phương trình $$\log_4(x+7)>\log_2(x+1)$$
| \((-1;2)\) | |
| \((2;4)\) | |
| \((-3;2)\) | |
| \((5;+\infty)\) |
1 lời giải
Chọn phương án A.
Điều kiện: \(\begin{cases}
x+7>0\\ x+1>0
\end{cases}\Leftrightarrow x>-1\) (1)
Ta có $$\begin{aligned}
&\,\log_4(x+7)>\log_2(x+1)\\
\Leftrightarrow&\,\dfrac{1}{2}\log_2(x+7)>\log_2(x+1)\\
\Leftrightarrow&\,\log_2\sqrt{x+7}>\log_2(x+1)\\
\Leftrightarrow&\,\sqrt{x+7}>x+1\\
\Leftrightarrow&\,x+7>(x+1)^2\\
\Leftrightarrow&\,x^2+x-6<0.
\end{aligned}$$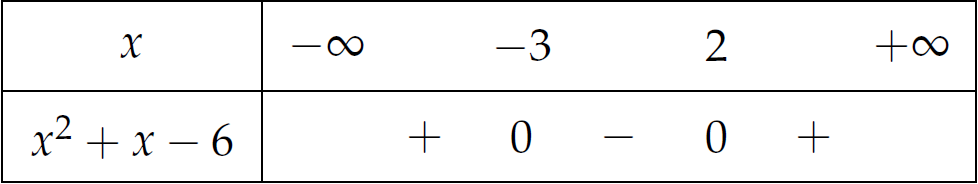
Suy ra \(x\in(-3;2)\) (2)
Từ (1) và (2) suy ra \(x\in(-1;2)\).
