Ngân hàng bài tập
A
Bất phương trình \(\dfrac{1}{x-1}+\dfrac{2}{x-2}>0\) có tập nghiệm là
| \(\left(1;\dfrac{4}{3}\right]\cup(2;+\infty)\) | |
| \(\left(1;\dfrac{4}{3}\right)\cup(2;+\infty)\) | |
| \((-\infty;1)\cup\left[\dfrac{4}{3};2\right)\) | |
| \(\left(\dfrac{4}{3};2\right)\cup(-\infty;1)\) |
1 lời giải
Chọn phương án B.
\(\begin{aligned}
\dfrac{1}{x-1}+\dfrac{2}{x-2}>0\Leftrightarrow&\dfrac{(x-2)+2(x-1)}{(x-1)(x-2)}>0\\
\Leftrightarrow&\dfrac{3x-4}{(x-1)(x-2)}>0.
\end{aligned}\)
- \(3x-4=0\Leftrightarrow x=\dfrac{4}{3}\).
- \(x-1=0\Leftrightarrow x=1\).
- \(x-2=0\Leftrightarrow x=2\).
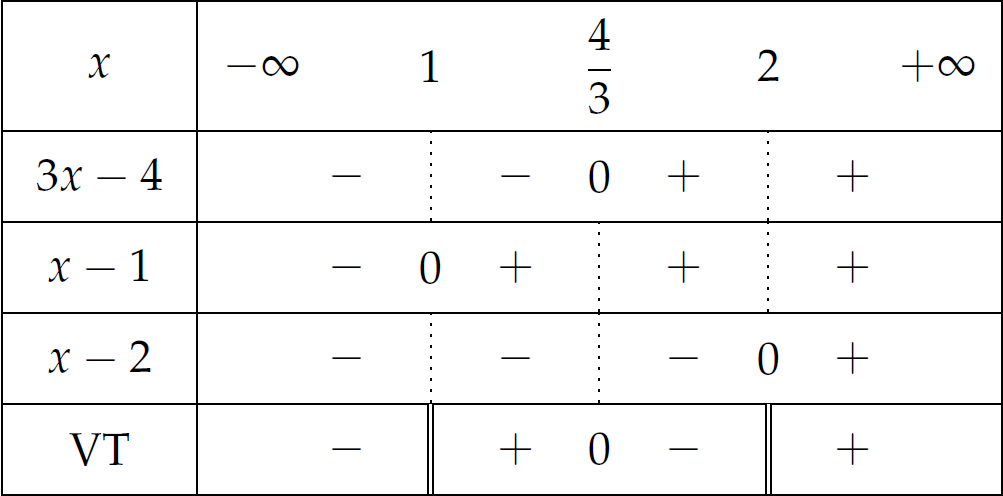
Suy ra \(S=\left(1;\dfrac{4}{3}\right)\cup(2;+\infty)\).
