Ngân hàng bài tập
C
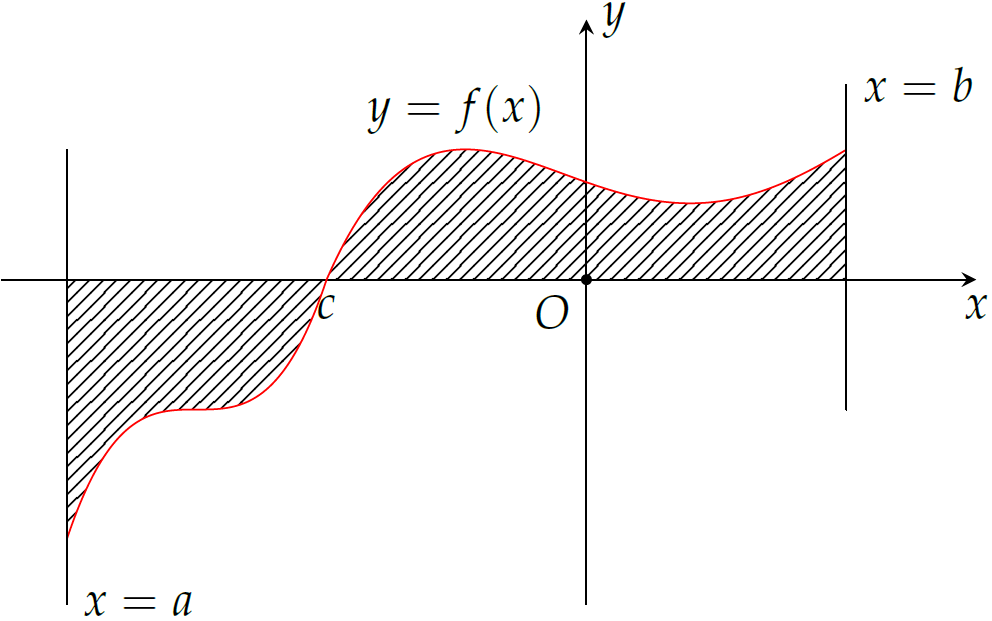
Diện tích hình phẳng \((H)\) giới hạn bởi đồ thị của hàm số \(y=f(x)\), trục hoành và hai đường thẳng \(x=a\), \(x=b\) (\(a<b\) và \(f(x)\) liên tục trên \([a;b]\)) (phần gạch sọc trong hình vẽ) tính theo công thức
| \(S=-\displaystyle\int\limits_{a}^{c}f(x)\mathrm{\,d}x+\displaystyle\int\limits_{c}^{b}f(x)\mathrm{\,d}x\) | |
| \(S=\displaystyle\int\limits_{a}^{b}f(x)\mathrm{\,d}x\) | |
| \(S=\left|\displaystyle\int\limits_{a}^{b}f(x)\mathrm{\,d}x\right|\) | |
| \(S=\displaystyle\int\limits_{a}^{c}f(x)\mathrm{\,d}x+\displaystyle\int\limits_{c}^{b}f(x)\mathrm{\,d}x\) |
1 lời giải
Chọn phương án A.
\(\begin{aligned}
S&=\displaystyle\int\limits_{a}^{b}\left|f(x)\right|\mathrm{\,d}x\\
&=-\displaystyle\int\limits_{a}^{c}f(x)\mathrm{\,d}x+\displaystyle\int\limits_{c}^{b}f(x)\mathrm{\,d}x.
\end{aligned}\)
