Ngân hàng bài tập
B
Gọi \(M,\,m\) lần lượt là giá trị lớn nhất và giá trị nhỏ nhất của hàm số \(y=\sin x+\cos x\). Tính \(P=M-m\).
| \(P=4\) | |
| \(P=2\sqrt{2}\) | |
| \(P=\sqrt{2}\) | |
| \(P=2\) |
2 lời giải
Chọn phương án B.
Dùng chức năng TABLE của máy tính cầm tay
- Đưa máy tính về đơn vị độ (DEG) cho dễ chọn bước nhảy
- Chọn chế độ một hàm \(f(x)\) trong TABLE

- Nhập hàm số \(f(x)=\sin x+\cos x\)

- Chọn bước nhảy thích hợp với cấu hình của máy
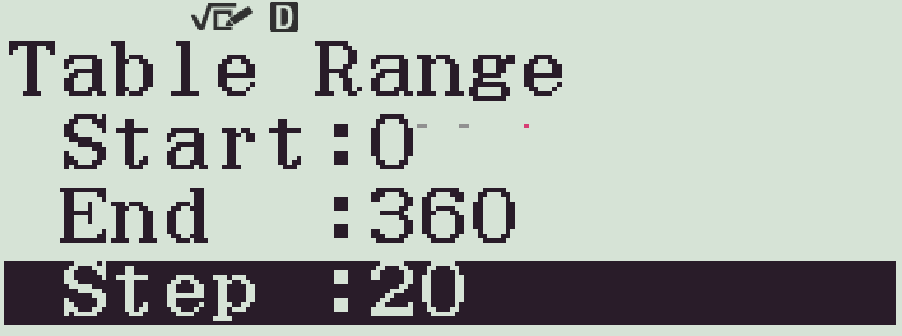
- Quan sát, tìm GTLN và GTNN
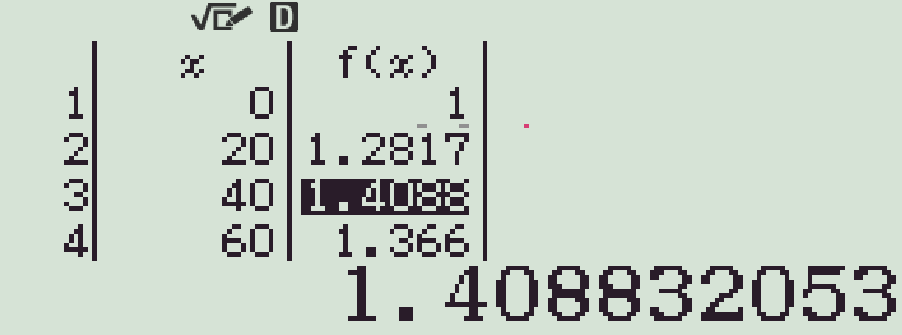
Theo đó \(M\approx\sqrt{2}\), \(m\approx-\sqrt{2}\).
Suy ra \(P=M-m=2\sqrt{2}\).
Chọn phương án B.
Ta có \(y=\sin x+\cos x=\sqrt{2}\sin\left(x+\dfrac{\pi}{4}\right)\).
$$\begin{eqnarray*}
-1\leq&\sin\left(x+\dfrac{\pi}{4}\right)&\leq1\\
\Leftrightarrow-\sqrt{2}\leq&\sqrt{2}\sin\left(x+\dfrac{\pi}{4}\right)&\leq\sqrt{2}\\
\Leftrightarrow-\sqrt{2}\leq&y&\leq\sqrt{2}.
\end{eqnarray*}$$
Vậy \(\begin{cases}
M=\sqrt{2}\\ m=-\sqrt{2}
\end{cases}\Rightarrow P=M-m=2\sqrt{2}\).
Vận dụng công thức cộng ta có:
- \(\sin x+\cos x=\sqrt{2}\sin\left(x+\dfrac{\pi}{4}\right)\)
- \(\sin x-\cos x=\sqrt{2}\sin\left(x-\dfrac{\pi}{4}\right)\)
