Ngân hàng bài tập
S
Cho biểu thức \(f(x)=\dfrac{(x-3)(x+2)}{x^2-1}\). Có tất cả bao nhiêu giá trị nguyên âm của \(x\) thỏa mãn \(f(x)<1\)?
| \(1\) | |
| \(2\) | |
| \(3\) | |
| \(4\) |
1 lời giải
Chọn phương án C.
\(\begin{eqnarray*}
&f(x)&<1\\
\Leftrightarrow&\dfrac{(x-3)(x+2)}{x^2-1}&<1\\
\Leftrightarrow&\dfrac{x^2-x-6}{x^2-1}-1&<0\\
\Leftrightarrow&\dfrac{x^2-x-6-\left(x^2-1\right)}{x^2-1}&<0\\
\Leftrightarrow&\dfrac{-x-5}{(x-1)(x+1)}&<0
\end{eqnarray*}\)
Bảng xét dấu: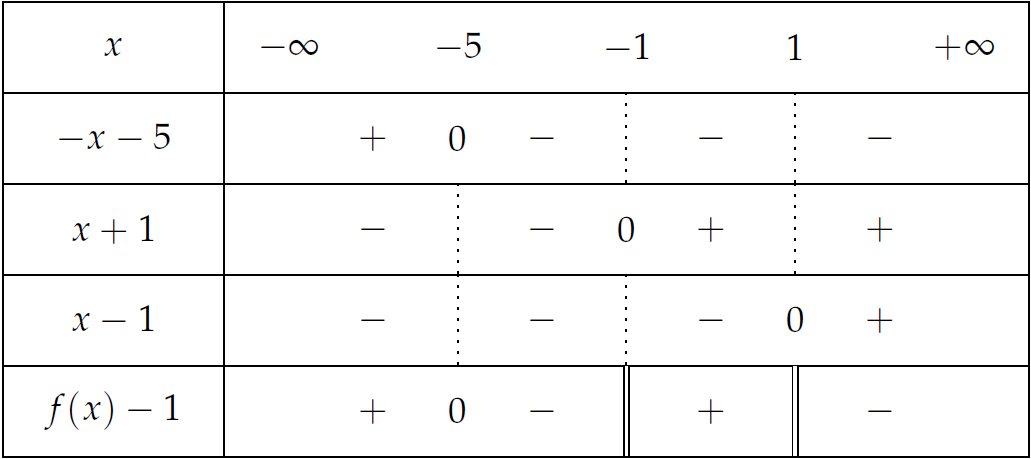
Theo đó, \(f(x)-1<0\Leftrightarrow x\in(-5;-1)\cup(1;+\infty)\).
Vậy có \(3\) giá trị nguyên âm thỏa đề là \(-4;-3;-2\).
